
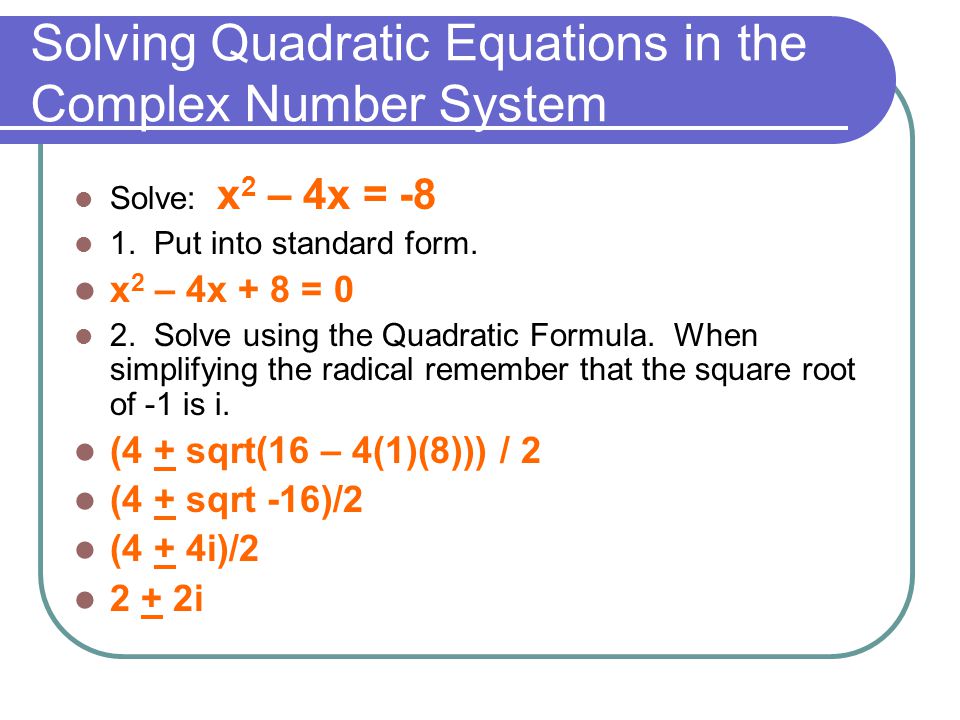
Step 2 - Rearrange the equations so that terms depending on the unknowns \( i_1, i_2 \) and \( i_3 \) are on the left and all constants on the right, and set the unknowns in the same order in all equations: Step 2: Use Kirchhoff's laws of currents to write an equation at each node: We denote -1 with the symbol ‘i’, which denotes Iota (Imaginary number). Thus, with the introduction of complex numbers, we have Imaginary roots. The calculator also converts a complex number into angle notation (phasor notation. As an imaginary unit, use i or j (in electrical engineering), which satisfies the basic equation i2 1 or j2 1. The roots of the equation are of form x -1 and no real roots exist. This calculator does basic arithmetic on complex numbers and evaluates expressions in the set of complex numbers. Loop L1: \( v_i - Z_1 i_1 - Z_2 i_2 = 0 \) The complex numbers were introduced to solve the equation x 2 +1 0. Step 1 - Use Kirchhoff's laws of voltages to write an equation for each closed loop: Write the voltages and Currents Equations Using Kirchhoff's and Solve ExampleĪn example of 3 equations is done for you and you may follow it at the start before using the calculator for other circuits that may involve any number of equations.įind the currents through the impedances in the circuit below, given the voltage sources \( v_i = 9 \angle 0 \) Volts, \( Z_1 = 15 + j 10 \ \Omega \), \( Z_2 = 4.8 - j 3.6 \ \Omega \), \( Z_3 = - j5 \ \Omega \) and \( Z_4 = 7.5 + j 7.5 \ \Omega \). A complex number is a number that can be expressed in the form a + bi where 'a' and 'b' are real numbers and 'i' is the imaginary unit, which satisfies the equation i 2 -1. The outputs are the values of all currents in the given circuit. The calculator accepts any number of equations. The following operations can be performedĢ*x - multiplication 3/x - division x^2 - squaring x^3 - cubing x^5 - raising to the power x + 7 - addition x - 6 - subtraction Real numbers insert as 7.AC Kirchhoff's Equations Solver and Calculator AC Kirchhoff's Equations Solver and CalculatorĪn online calculator to solve Kirchhoff's equations in ac circuits, using complex numbers, is presented. The error function erf(x) (integral of probability), Hyperbolic cosecant csch(x), hyperbolic arcsecant asech(x),
Secant sec(x), cosecant csc(x), arcsecant asec(x),Īrccosecant acsc(x), hyperbolic secant sech(x), The key feature of our calculator is that each determinant can be calculated apart and you can also check the exact type of matrix if the determinant of the main matrix is zero.
#SYSTEM OF EQUATIONS SOLVER WITH COMPLEX NUMBERS FOR FREE#
Other trigonometry and hyperbolic functions: Here you can solve systems of simultaneous linear equations using Cramer's Rule Calculator with complex numbers online for free with a very detailed solution.

Hyperbolic arctangent atanh(x), hyperbolic arccotangent acoth(x) Hyperbolic arcsine asinh(x), hyperbolic arccosinus acosh(x), Hyperbolic tangent and cotangent tanh(x), ctanh(x) Hyperbolic sine sh(x), hyperbolic cosine ch(x), Sinus sin(x), cosine cos(x), tangent tan(x), cotangent ctan(x)Įxponential functions and exponents exp(x)Īrcsine asin(x), arccosine acos(x), arctangent atan(x), The modulus or absolute value: absolute(x) or |x|


 0 kommentar(er)
0 kommentar(er)
